Differential Equations: Verification

Verification of a differential equation is exactly like plugging in the last piece of a jigsaw puzzle; the solution is inserted back into the differential equation to ensure that it qualifies its conditions. Apart from the satisfaction of finding out a successful solution that may have taken hours of effort, learning to verify accurately is also important for various other reasons. Fortunately, verification is far simpler than actually solving most differential equations.

HOW DO YOU VERIFY A DIFFERENTIAL EQUATION? 

The verification of a solution for a differential equation would mostly require basic knowledge of differentiation and integration, the two pillars of calculus. In general, differentiation is related to finding the slope/gradient of a curve at a particular point, while integration is associated with finding the area underneath the curve between 2 points. For a complete revision, refer to this external source.

                                         

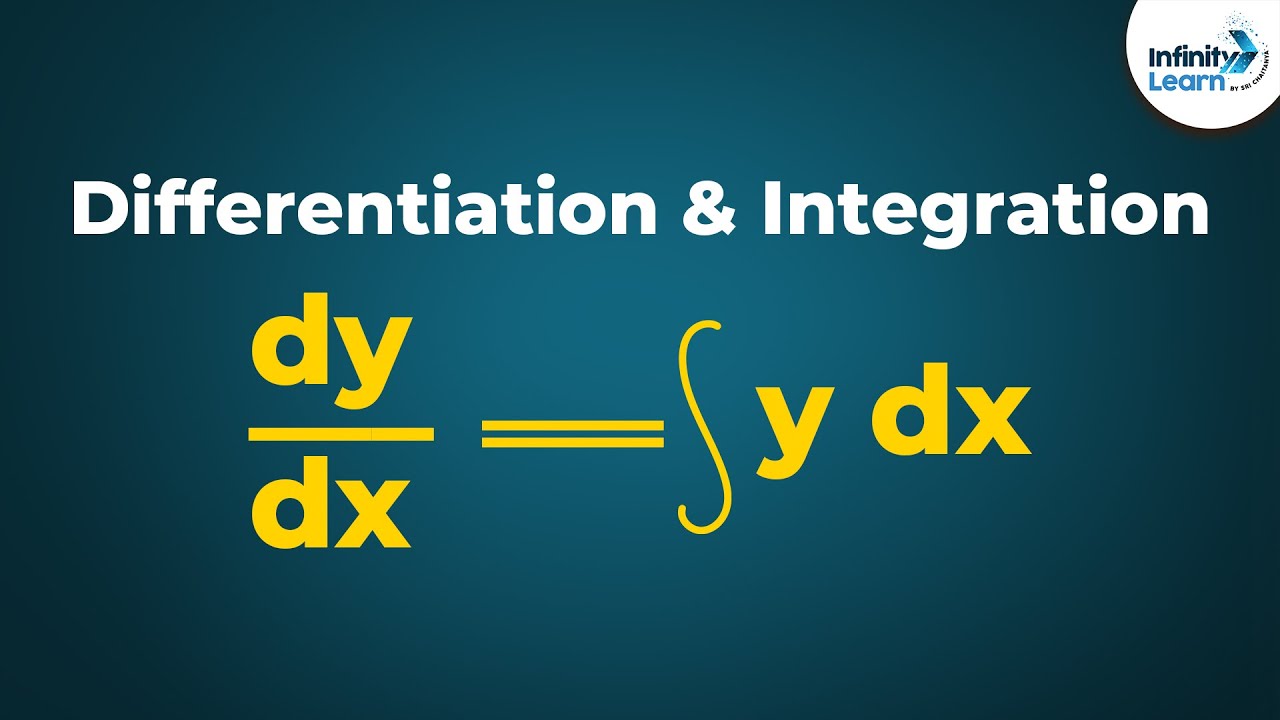
The verification simply involves using the given solution and processing the variables required by the equation from this solution. Finally, the variables in the equation need to be plugged in with these calculated values and the equal relation can be confirmed. This is enough to verify the solution; however, it provides no proof that it is the only solution.

In the next post, we will start the process of actually solving differential equations, a prospect that scares most people. While solving differential equations can get very complicated, sometimes the differential equation can be solved as easily as the one below. [The equation below does qualify as a differential equation since it works with derivatives of a function].

Nevertheless, the main lesson that needs to be taken away from this post is that verification is an important part of differential equations, since it provides us with a far better understanding of how differential equations behave. This experience can help to spark inspiration for faster solving methods. Learn more about differential equations through this oversimplified presentation.